KJHの博客

记录&回忆

0%

数据结构BFS、DFS

发表于 更新于 分类于
本文字数： 3.6k 阅读时长 ≈ 3 分钟

这里则介绍图的另外一种存储方式：邻接矩阵。参考资料《大话数据结构》《C算法：卷二》

一、图的数据结构

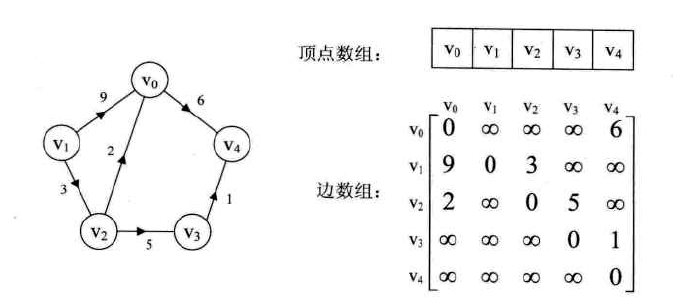
图的邻接矩阵存储方式是用两个数据来表示。一个一维数组存储图中顶点信息，一个二维数组（称为邻接矩阵）存储图中的边的信息。

见下图：（图片来源于《大话数据结构》）

​ img

1
2
3
4
5
6
7
8
9
10
11
12
/*图的邻接矩阵存储*/
typedef int VertexType;
typedef int EdgeType;
#define MAXVEX 100
#define INFI 65535
typedef struct 
{
	VertexType vexs[MAXVEX];           /*顶点表*/
	EdgeType matrix[MAXVEX][MAXVEX];   /*邻接矩阵*/
	unsigned int numVertexes;          /*顶点数*/
	unsigned int numEdges;             /*边数*/
}Graph;

二、创建一个图

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
/*创建一个邻接矩阵无向图*/
Graph* CreateGraph()
{
	Graph *pGragh = new Graph;
	if (NULL == pGragh)
		return NULL;
	cout << "输入顶点数和边数：" << endl;
	cin >> pGragh->numVertexes >> pGragh->numEdges;
	for (int i = 0; i < pGragh->numVertexes; ++i)/*建立顶点表*/
		(pGragh->vexs)[i] = i;
	for (int i = 0; i < pGragh->numVertexes; ++i)/*邻接矩阵初始化*/
	{
		for (int j = 0; j < pGragh->numVertexes; ++j)
		{
			(pGragh->matrix)[i][j] = INFI;
			if (i == j)
				(pGragh->matrix)[i][j] = 0;
		}
	}
	for (int k = 0; k < pGragh->numEdges; ++k)
	{
		int i, j, w;
		cout << "输入边(vi,vj)上的下标i，下标j和权重w：" << endl;
		cin >> i >> j >> w;
		(pGragh->matrix)[i][j] = w;
		(pGragh->matrix)[j][i] = (pGragh->matrix)[i][j];//无向图是对称矩阵
	}
	return pGragh;
}
/*创建一个邻接矩阵有向图*/
Graph* CreateDiGraph()
{
	Graph *pGragh = new Graph;
	if (NULL == pGragh)
		return NULL;
	cout << "输入顶点数和边数：" << endl;
	cin >> pGragh->numVertexes >> pGragh->numEdges;
	for (int i = 0; i < pGragh->numVertexes; ++i)/*建立顶点表*/
		(pGragh->vexs)[i] = i;
	for (int i = 0; i < pGragh->numVertexes; ++i)/*邻接矩阵初始化*/
	{
		for (int j = 0; j < pGragh->numVertexes; ++j)
		{
			(pGragh->matrix)[i][j] = INFI;
			if (i == j)
				(pGragh->matrix)[i][j] = 0;
		}
	}
	for (int k = 0; k < pGragh->numEdges; ++k)
	{
		int i, j, w;
		cout << "输入边<vi,vj>上的下标i，下标j和权重w：" << endl;
		cin >> i >> j >> w;
		(pGragh->matrix)[i][j] = w;//清楚上面输入的顺序，有向边的开始点和终点
	}
	return pGragh;
}

三、检查图中两个顶点间是否有边

1
2
3
4
5
6
7
8
9
10
/*检查两个顶点之间是否有边
对于邻接矩阵存储图，这比较简单。其中有向图对输入顶点顺序有要求*/
bool GraphHasEdge(Graph *pGraph, unsigned int begin, unsigned int end)
{
	if (NULL == pGraph || begin >= pGraph->numVertexes || end >= pGraph->numVertexes)
		return false;
	if (begin == end)
		return false;
	return ((pGraph->matrix)[begin][end] != INFI) ? true : false;
}

四、DFS

关于DFS与BFS的介绍见开篇链接

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
/*DFS*/
/*邻接矩阵的深度优先递归算法*/
void DFSUtil(Graph *pGraph, int start, bool visited[])
{
	visited[start] = true;
	cout << start << endl;
	for (int j = 0; j < pGraph->numVertexes; ++j)
	{
		if ((pGraph->matrix)[start][j] != 0 && (pGraph->matrix)[start][j] != INFI && !visited[j])
			DFSUtil(pGraph, j, visited);
	}
}
/*邻接矩阵的深度优先搜索*/
void DFS(Graph *pGraph)
{
	if (NULL == pGraph)
		return;
	bool *visited = new bool[pGraph->numVertexes];
	memset(visited, false, pGraph->numVertexes);
	for (int i = 0; i < pGraph->numVertexes; ++i)
	{
		if (!visited[i])
			DFSUtil(pGraph, i, visited);
	}
	delete[] visited;
}

五、BFS 

1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
/*BFS*/
void BFS(Graph *pGraph)
{
	if (NULL == pGraph)
		return;
	bool *visited = new bool[pGraph->numVertexes];
	memset(visited, false, pGraph->numVertexes);
	list<int> queue;//利用链表构造一个队列
	for (int i = 0; i < pGraph->numVertexes; ++i)
	{
		if (!visited[i])
		{
			visited[i] = true;
			cout << pGraph->vexs[i] << endl;
			queue.push_back(i);
			while (!queue.empty())
			{
				i = *queue.begin();
				queue.pop_front();
				for (int j = 0; j < pGraph->numVertexes; ++j)
				{
					if ((pGraph->matrix)[i][j] != 0 && (pGraph->matrix)[i][j] != INFI && !visited[j])
					{
						visited[j] = true;
						cout << pGraph->vexs[j] << endl;
						queue.push_back(j);
					}
				}
			}
		}
	}
}

这里只提供相关代码实现，代码已测试，理论部分请参考相关资料。